A (not too hard) coffee time puzzle this week. Go grab a pencil, a cup of coffee, and dust off a little geometery.
|
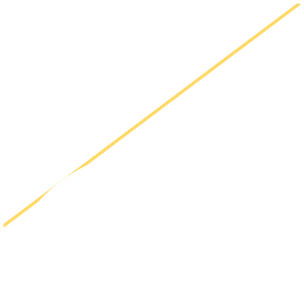|To the left is a unit square.A semi-circle is constructed inside the square with a diameter of one unit.The yellow line starts at the top corner, and is tangent to the semi-circle.What is the length of the yellow line?|
A semi-circle is constructed inside the square with a diameter of one unit.
|Show Solution|Give yourself five minutes, then push the solution button to check your answer.|
|First, let’s label the points:|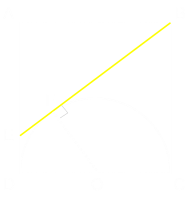
|
|
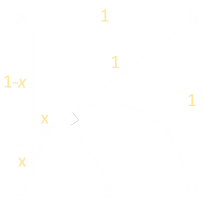|The length of each side of the square is one unit.The length BF is also one unit. If you are familiar with the Two Tanget Theorem, this is obvious straight away, if not, convince yourself this by imagining a right angle triangle BCO, where O is the center of the circle. The hypotenuse of this triangle OB, is the same as the hypotenuse of the similar triangle BFO. As OF and OC are also the same (being radii), then BF is also equal to BC.|
The length BF is also one unit. If you are familiar with the Two Tanget Theorem, this is obvious straight away, if not, convince yourself this by imagining a right angle triangle BCO, where O is the center of the circle. The hypotenuse of this triangle OB, is the same as the hypotenuse of the similar triangle BFO. As OF and OC are also the same (being radii), then BF is also equal to BC.
We’ll define x to be the distance EF.
Using the same tangent principle as above, this means that DE is also x, and therefore AE is equal to 1-x.
Using Pythagoras on triangle BAE:
Result
Now that we know *x, we can see that the length of BE = 11/4.*
You can find a complete list of all the articles here.
Click here to receive email alerts on new articles.